Transformation of Functions: Translation
Vertical Translation
For any function $f(x)$ and $c>0$, $f(x)+c$ vertically shifts the graph of $f(x)$ upward by $c$ units
and $f(x)-c$ vertically shifts the graph of $f(x)$ downward by $c$ units.
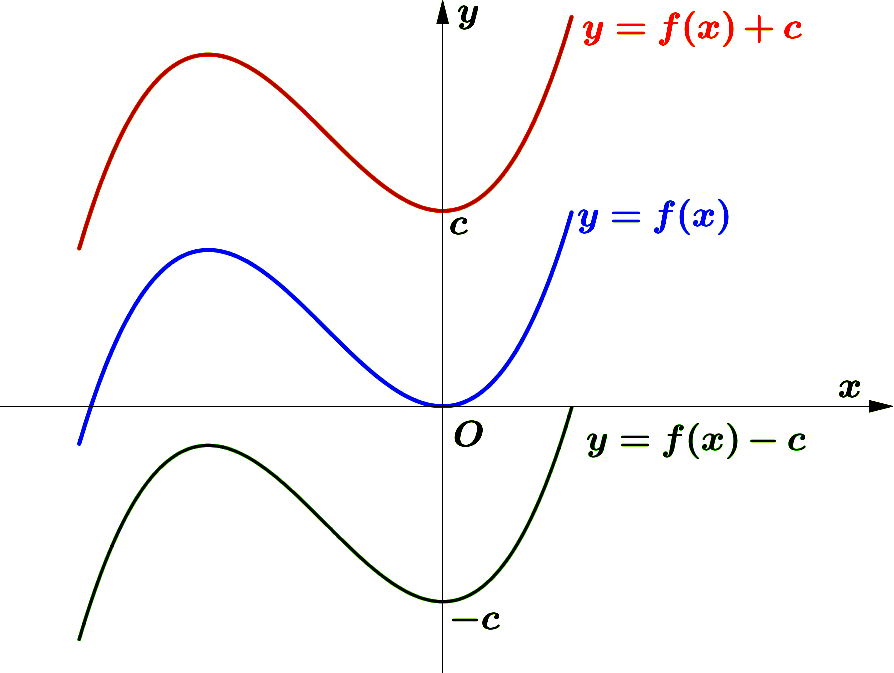
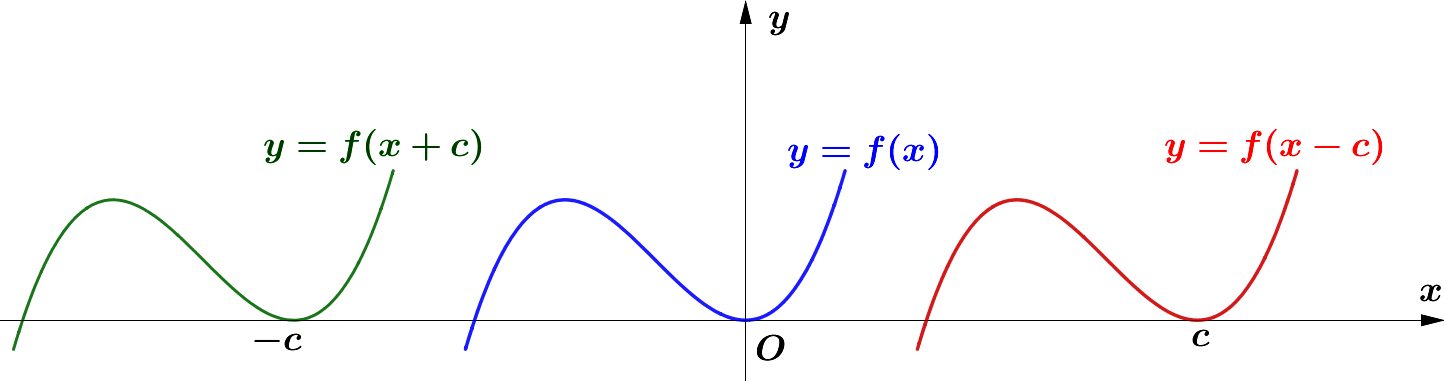
Horizontal Translation
For any function $f(x)$ and $c>0$, $f(x-c)$ horizontally shifts the graph of $f(x)$ right by $c$ units and
$f(x+c)$ horizontally shifts the graph of $f(x)$ left by $c$ units.
Question (1)
Use the graph of the function $f$ to sketch the graph of the following functions.
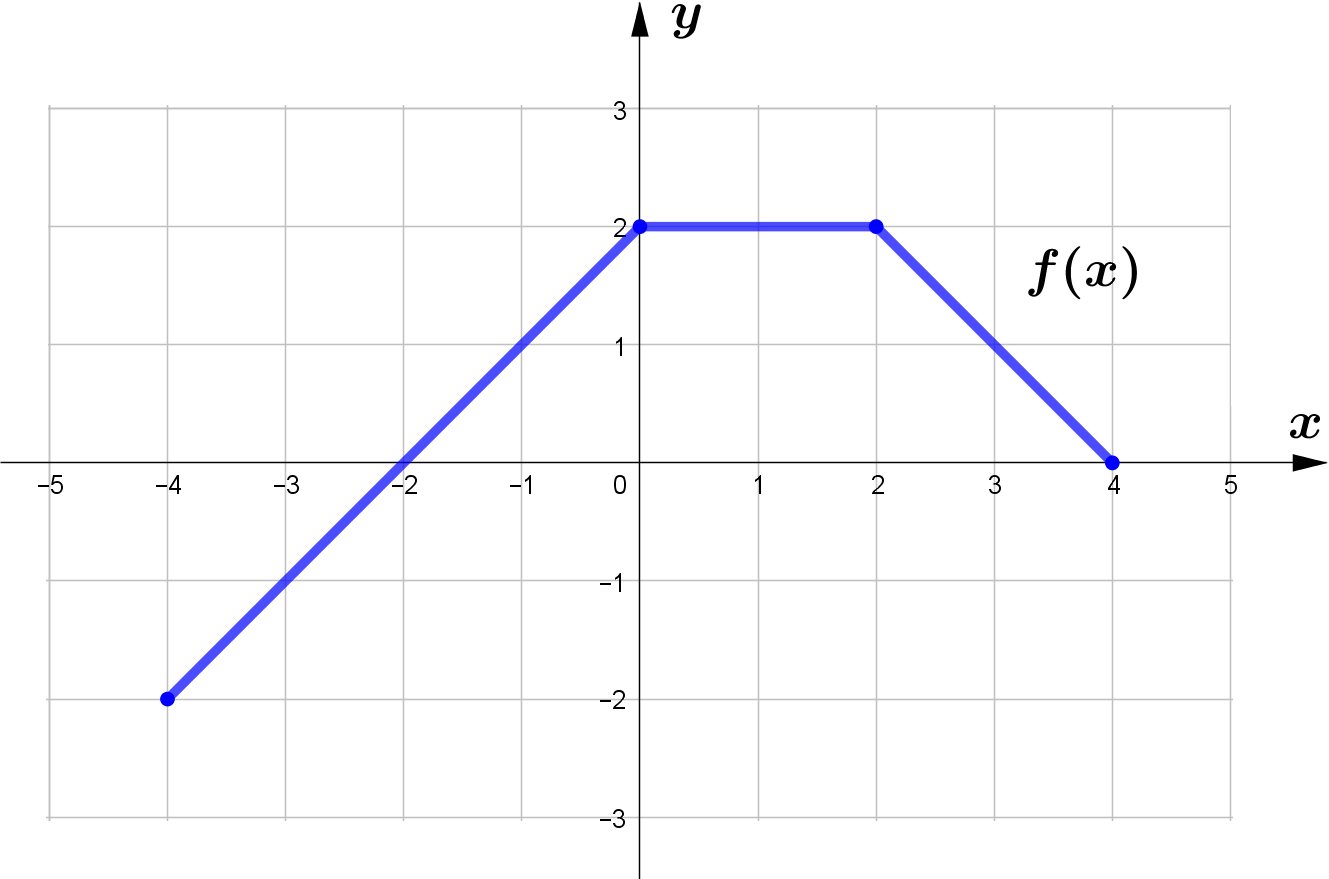
$\begin{array}{lll}
\text{(a) } g(x) = f(x) + 1 \\\\
\text{(b) } h(x) = f(x)-1 \\\\
\text{(c) } p(x) = f(x-1) \\\\
\text{(d) } F(x) = f(x+2) \\\\
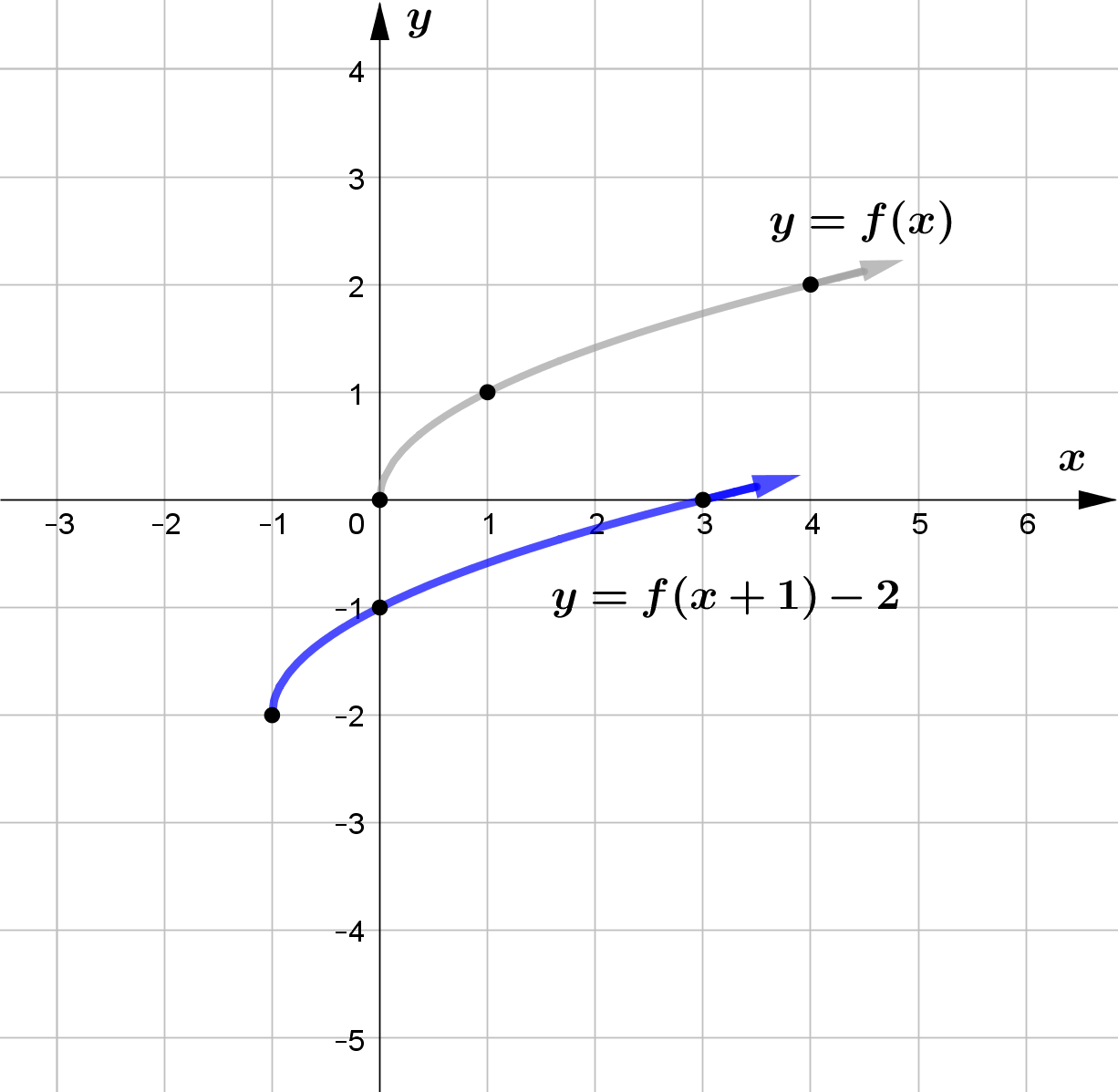
\text{(e) } G(x) = f(x+1) - 2\\\\
\text{(f) } H(x) = f(x-1) + 1
\end{array}$
SOLUTION
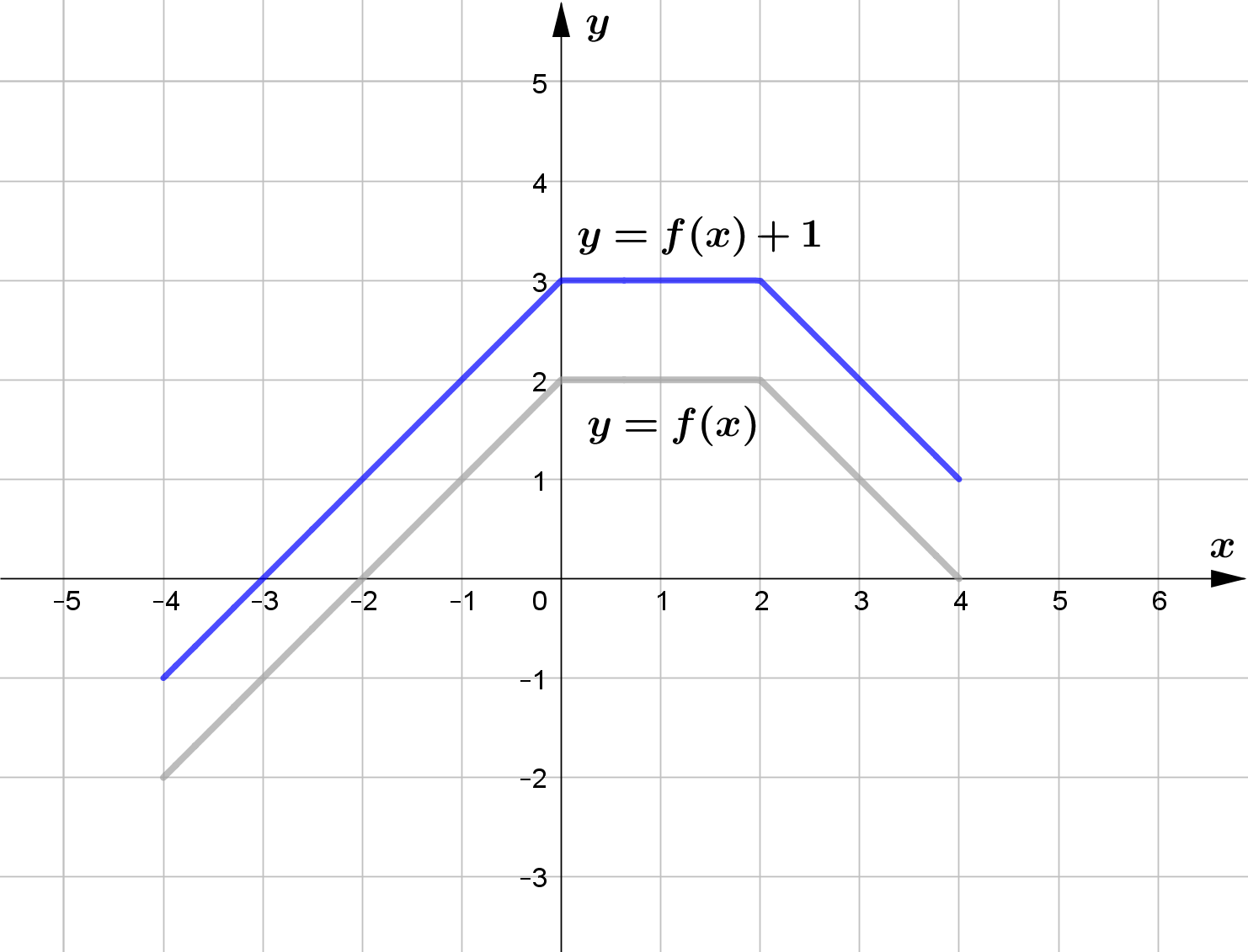
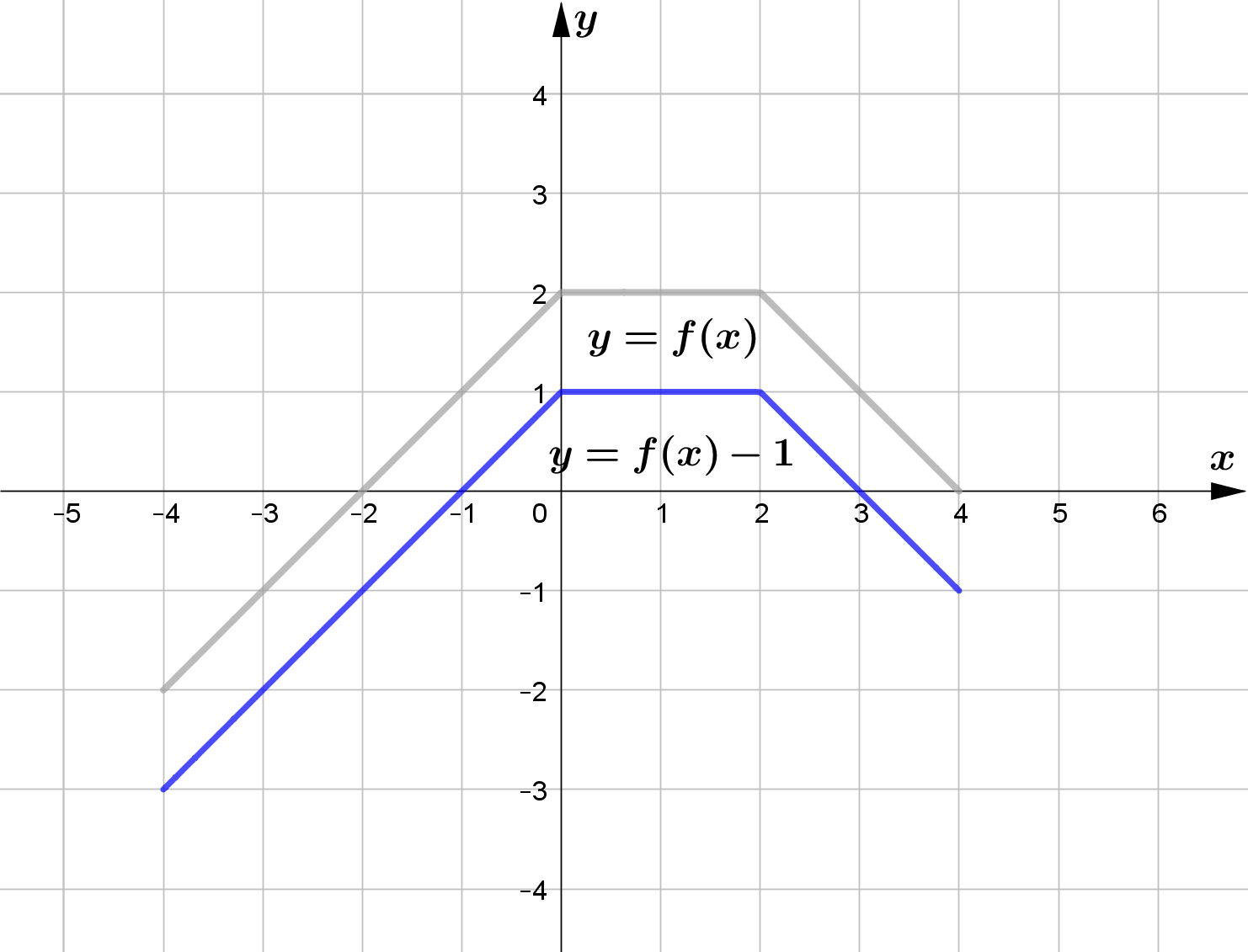
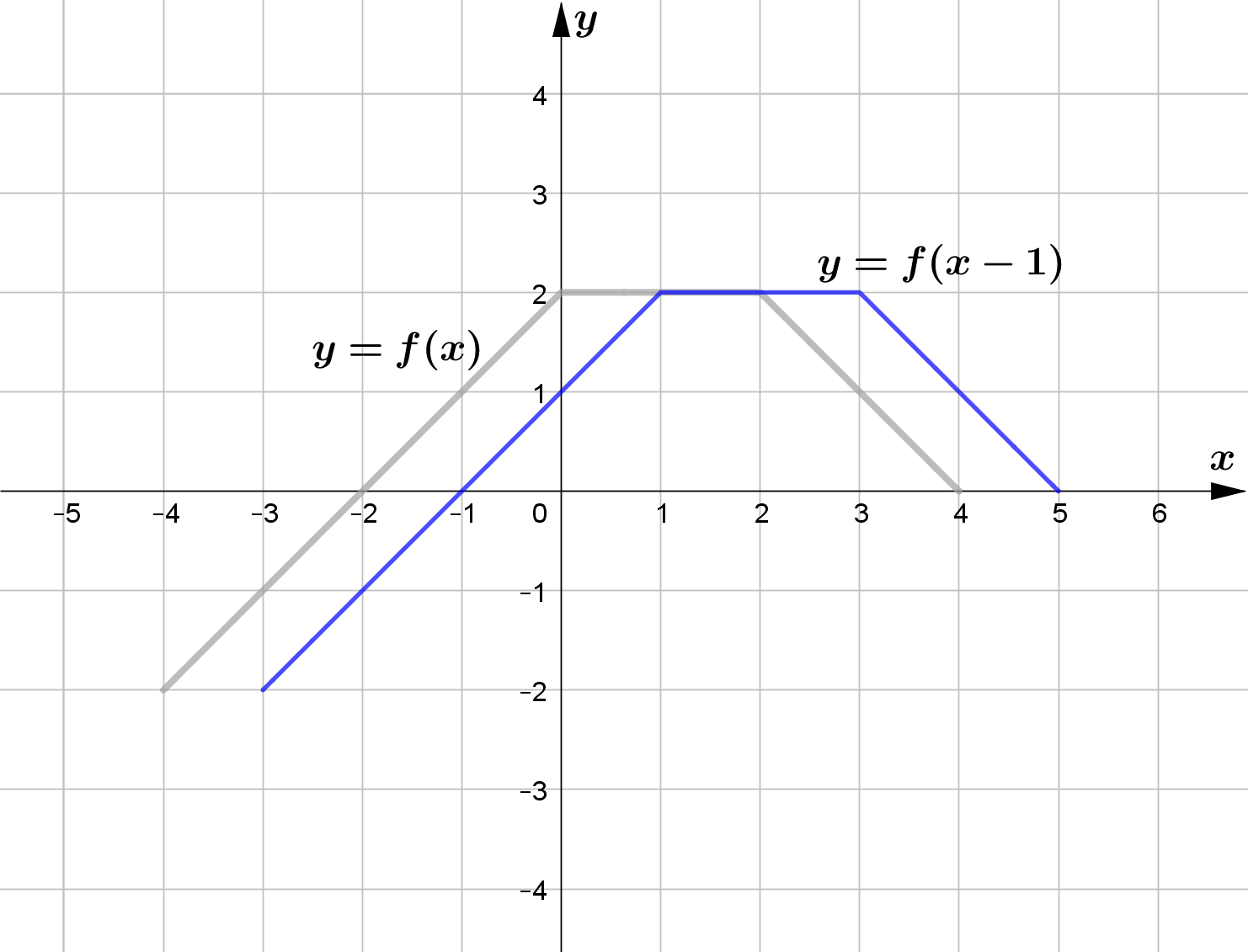
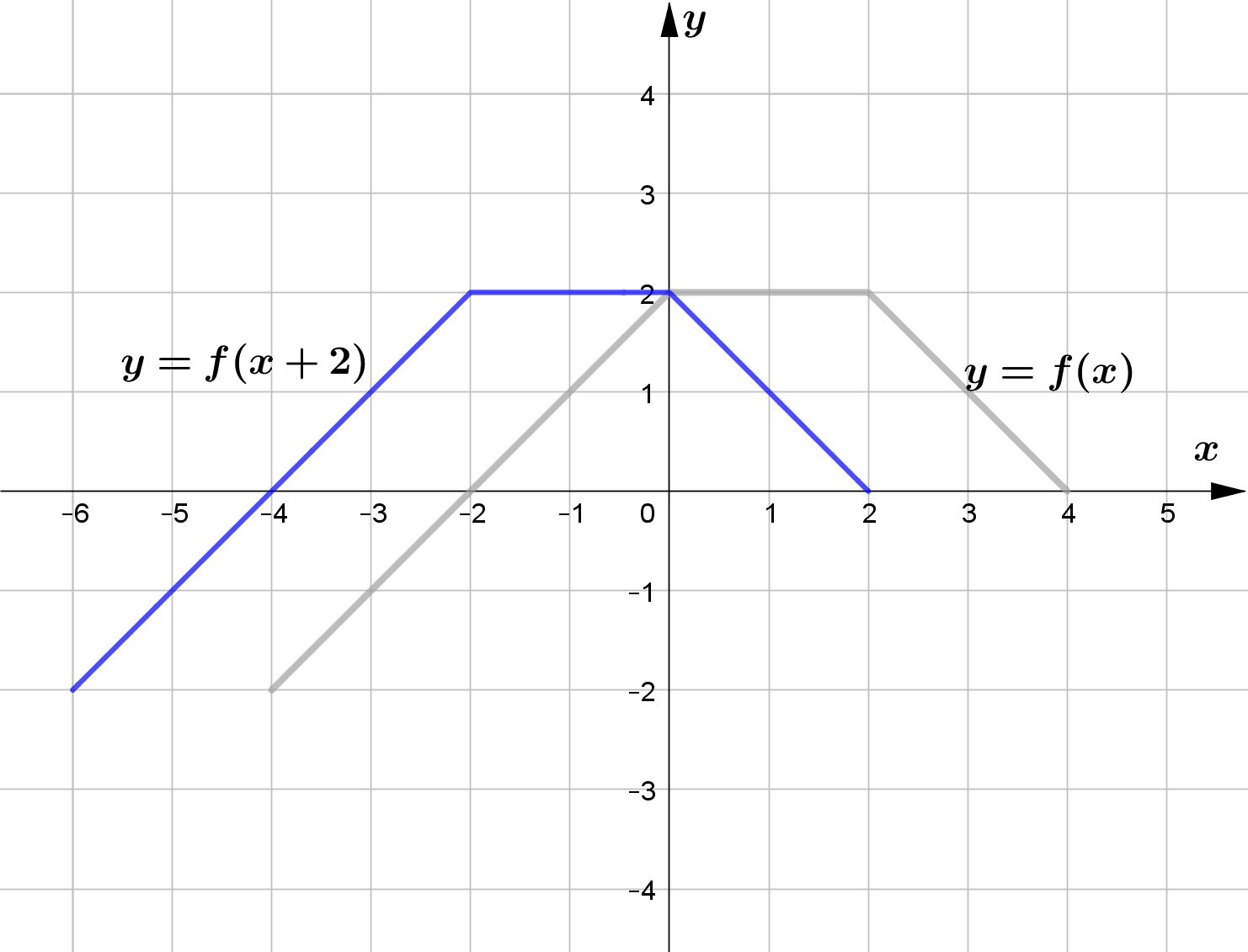
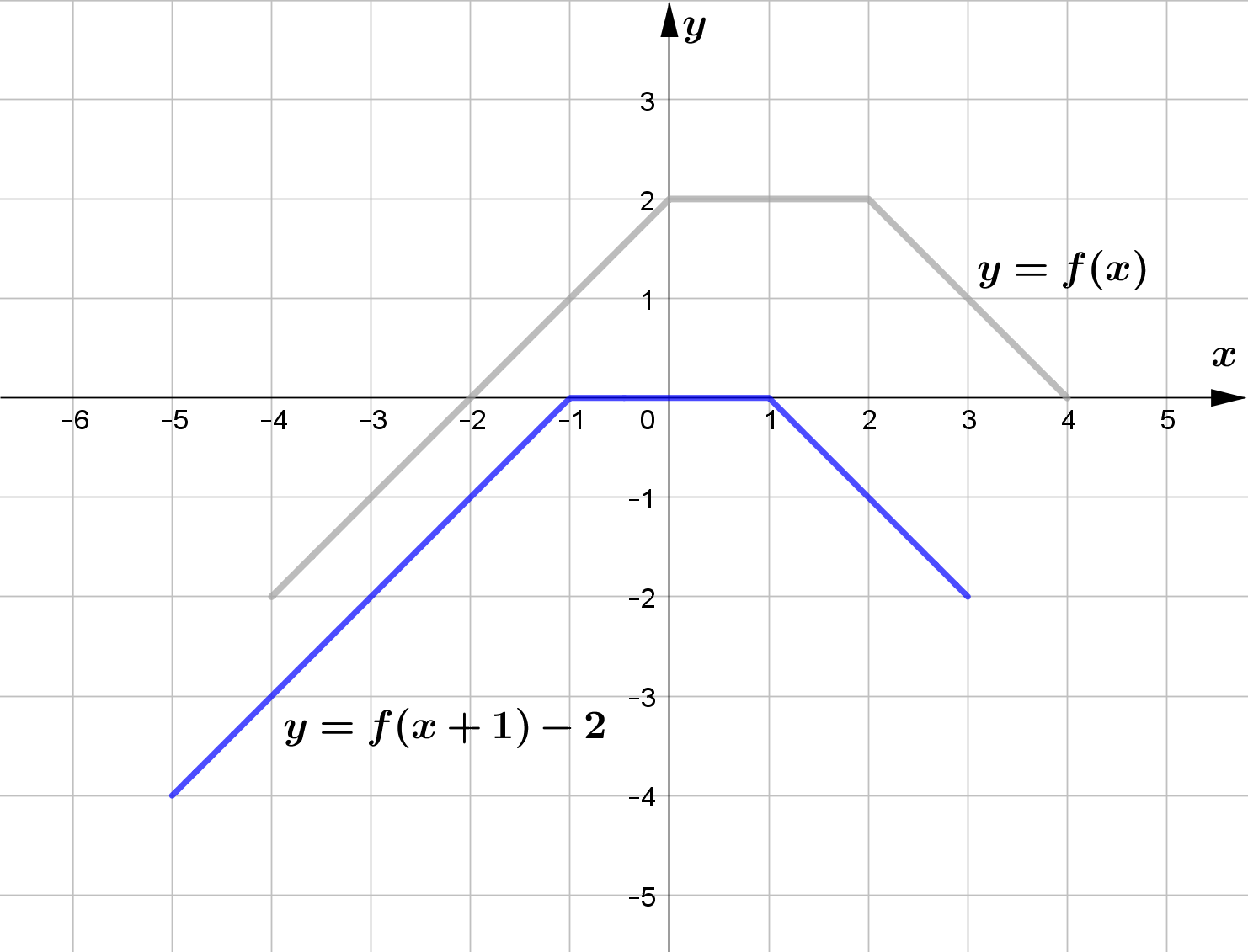
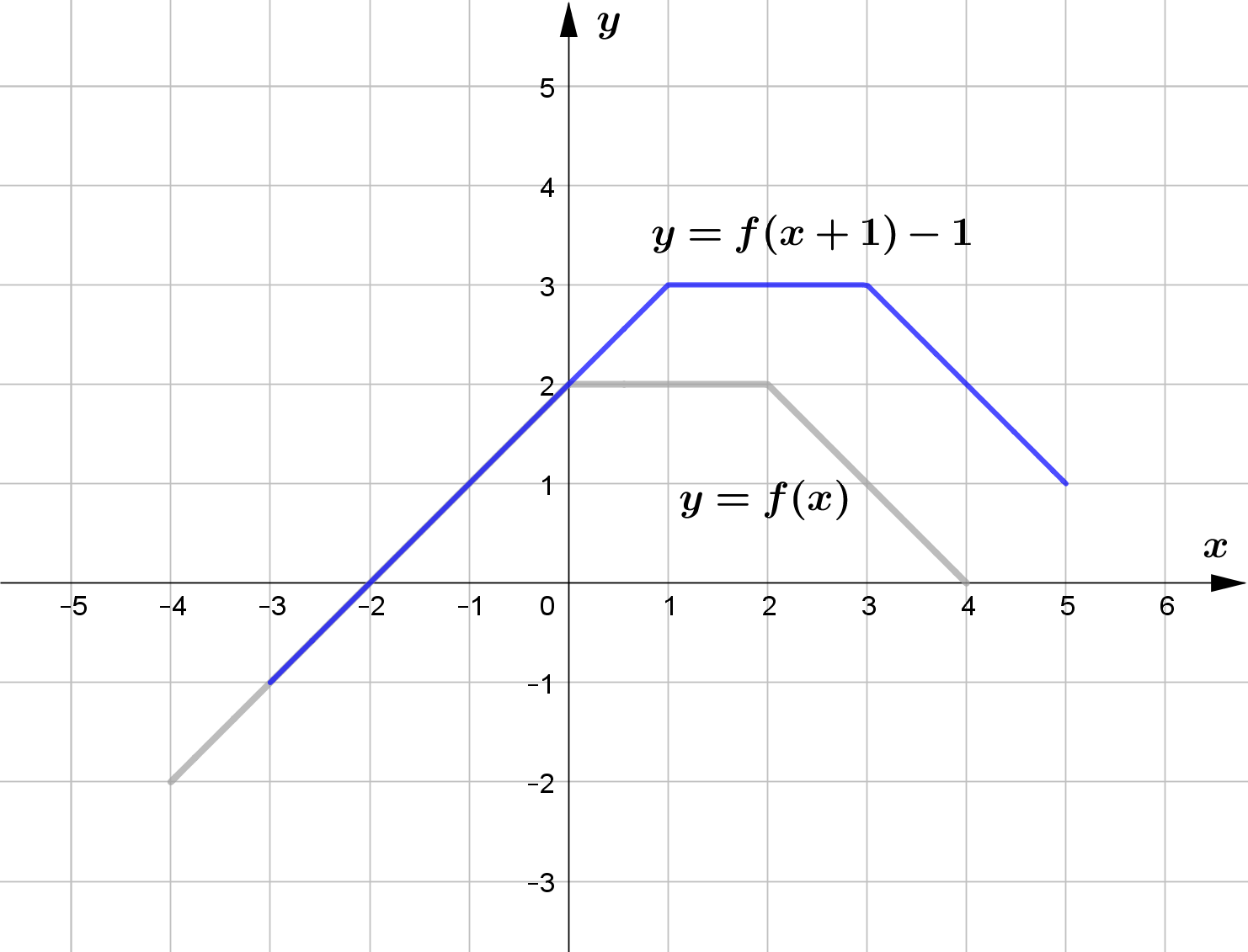 |
|---|
Question (2)
Draw the graph $f(x) = x^2$. Hence using the transformations of $f(x)$, draw the graph of the following functions.
$\begin{array}{lll}
\text{(a) } g(x) = f(x) + 1 \\\\
\text{(b) } h(x) = f(x) - 3 \\\\
\text{(c) } p(x) = f(x-1) \\\\
\text{(d) } F(x) = f(x+3) \\\\
\text{(e) } G(x) = f(x+1) - 2 \\\\
\text{(f ) } H(x) = f(x-2) + 3
\end{array}$
To sketch the graph of $y=x^2$, we should find some sample points on the graph.
$\begin{array}{|c|c|c|c|c|c|c|c|c|c|c|}
\hline x & -2 & -1 & 0 & 1 & 2\\
\hline y & 4 & 1 & 0 & 1 & 4\\
\hline
\end{array}$
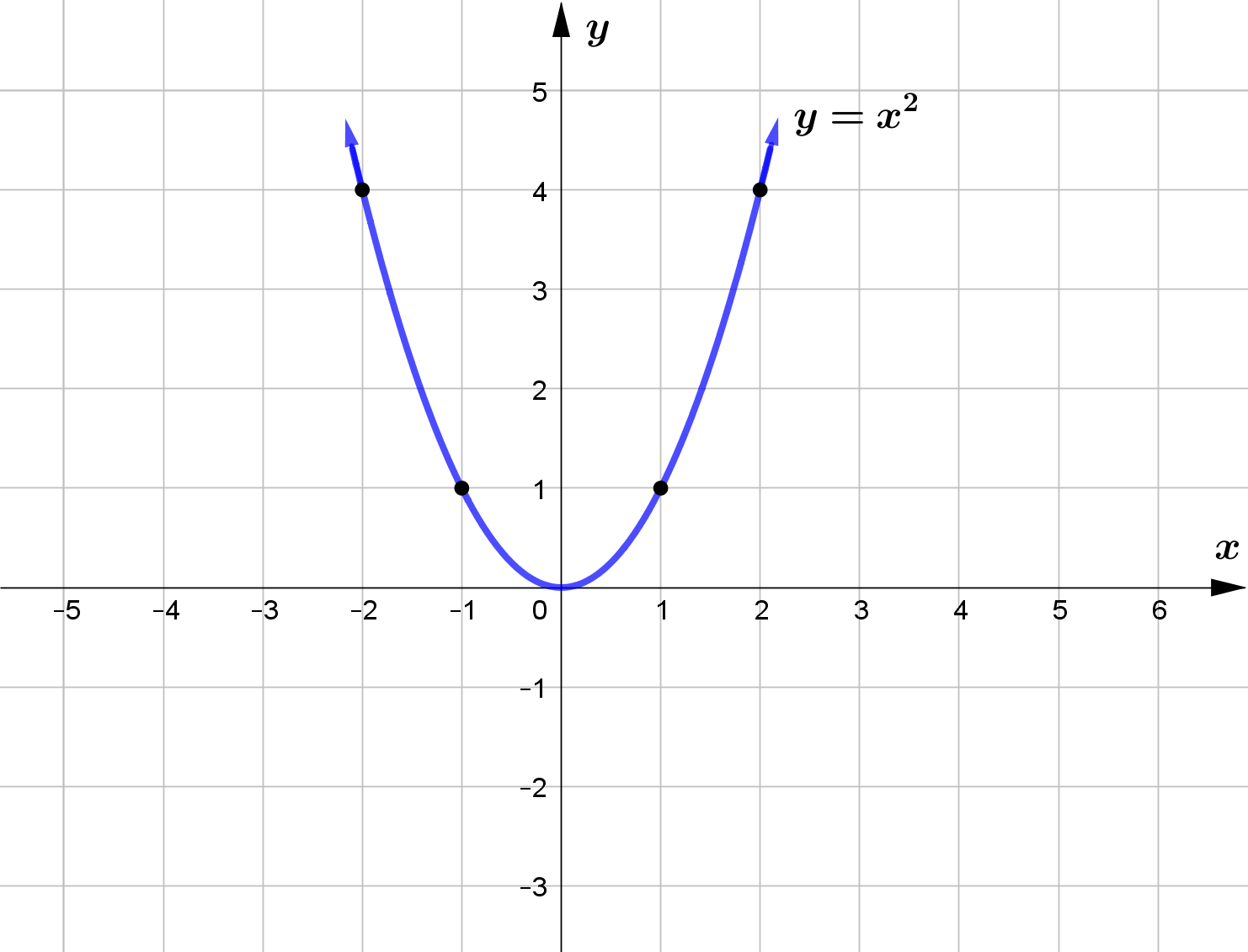
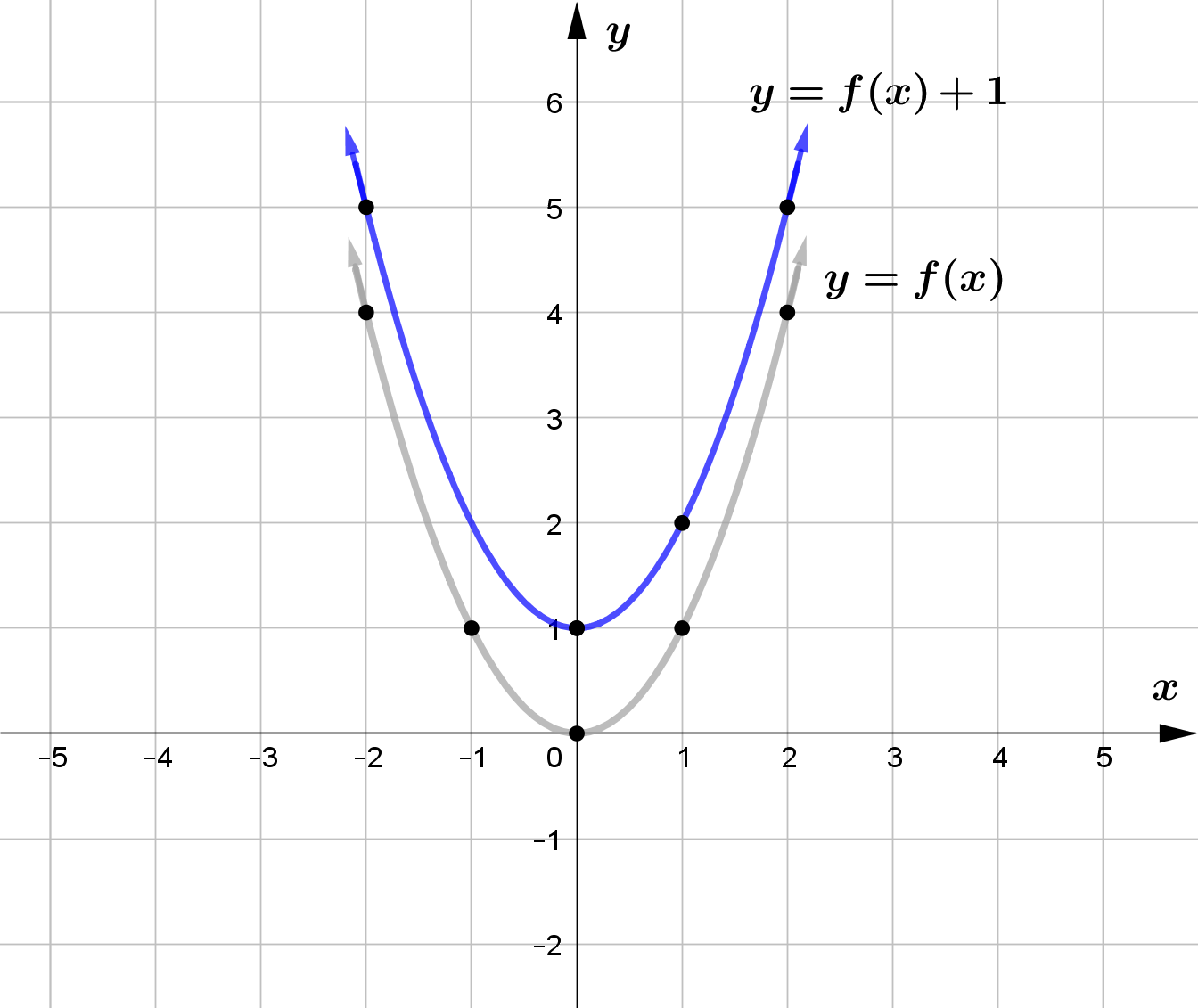
| (a) | $g(x)=f(x)+1$ |
| The grph of $y=g(x)$ can be obtained by shifting the grph of $y=f(x)$ 1 unit up. |
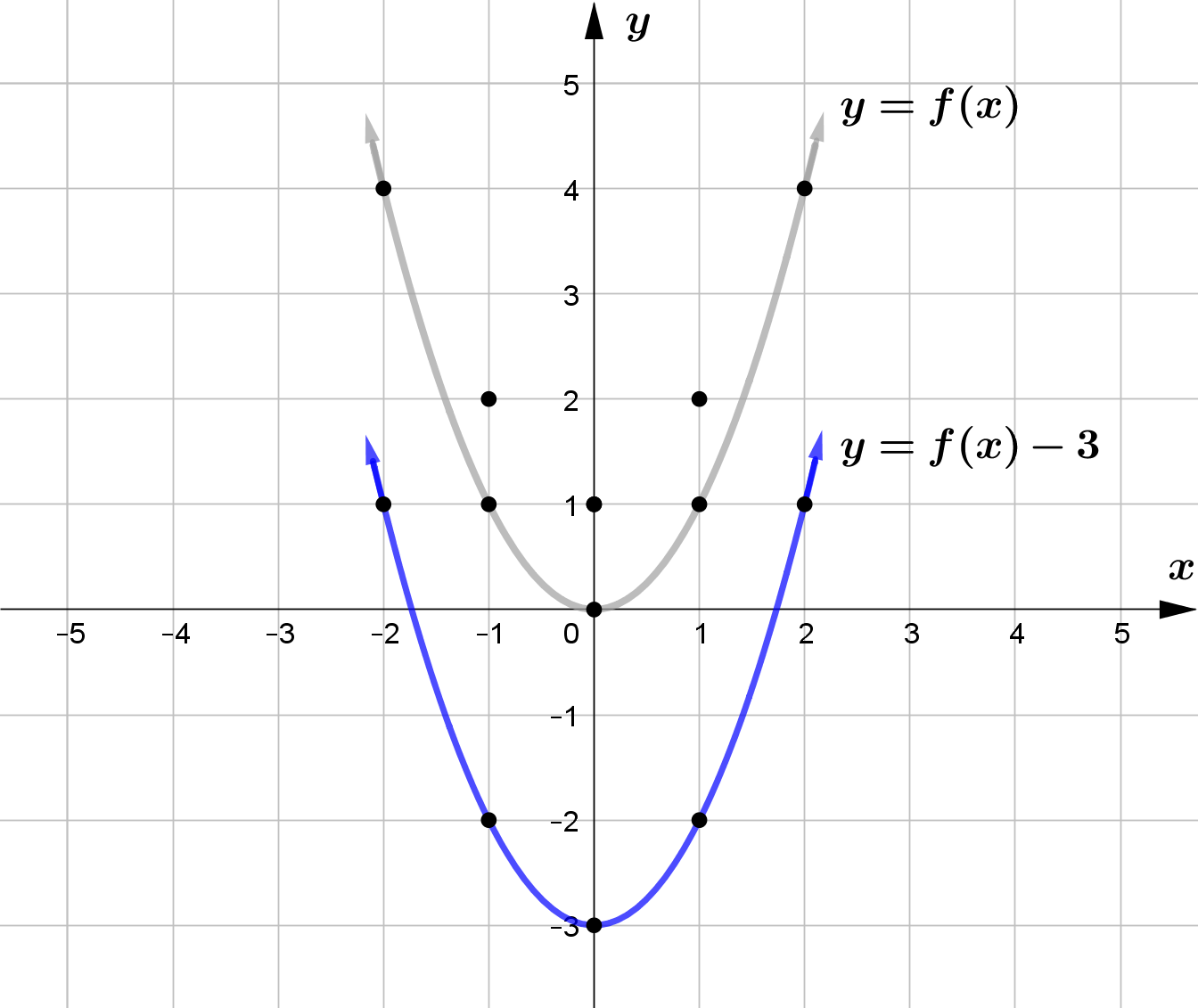
| (b) | $h(x)=f(x)−3$ |
| The grph of $y=h(x)$ can be obtained by shifting the grph of $y=f(x)$ 3 units down. |
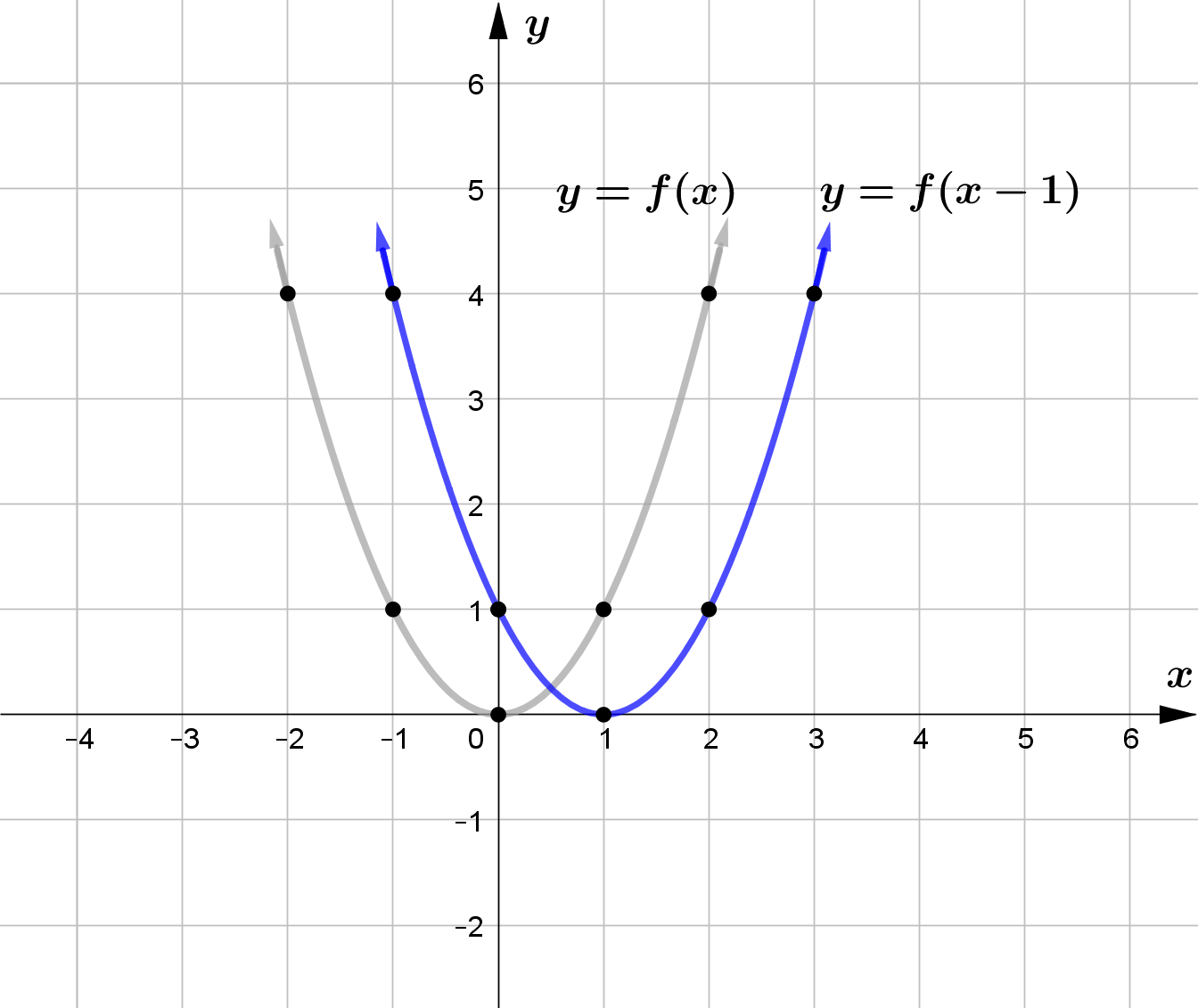
| (c) | $p(x)=f(x−1)$ |
| The grph of $y=p(x)$ can be obtained by shifting the grph of $y=f(x)$ 1 unit right. |
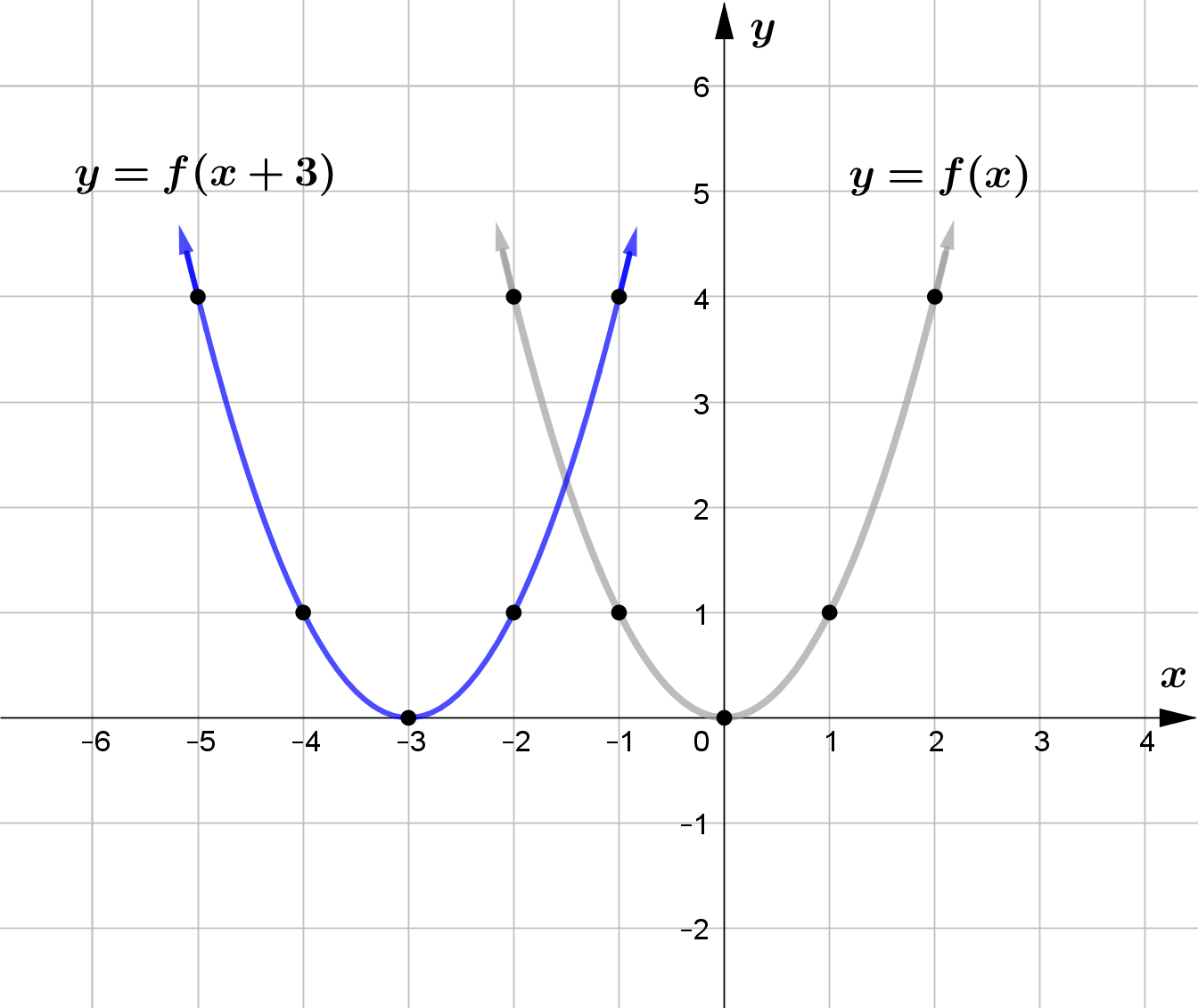
| (d) | $F(x)=f(x+3)$ |
| The grph of $y=F(x)$ can be obtained by shifting the grph of $y=f(x)$ 3 units left. |
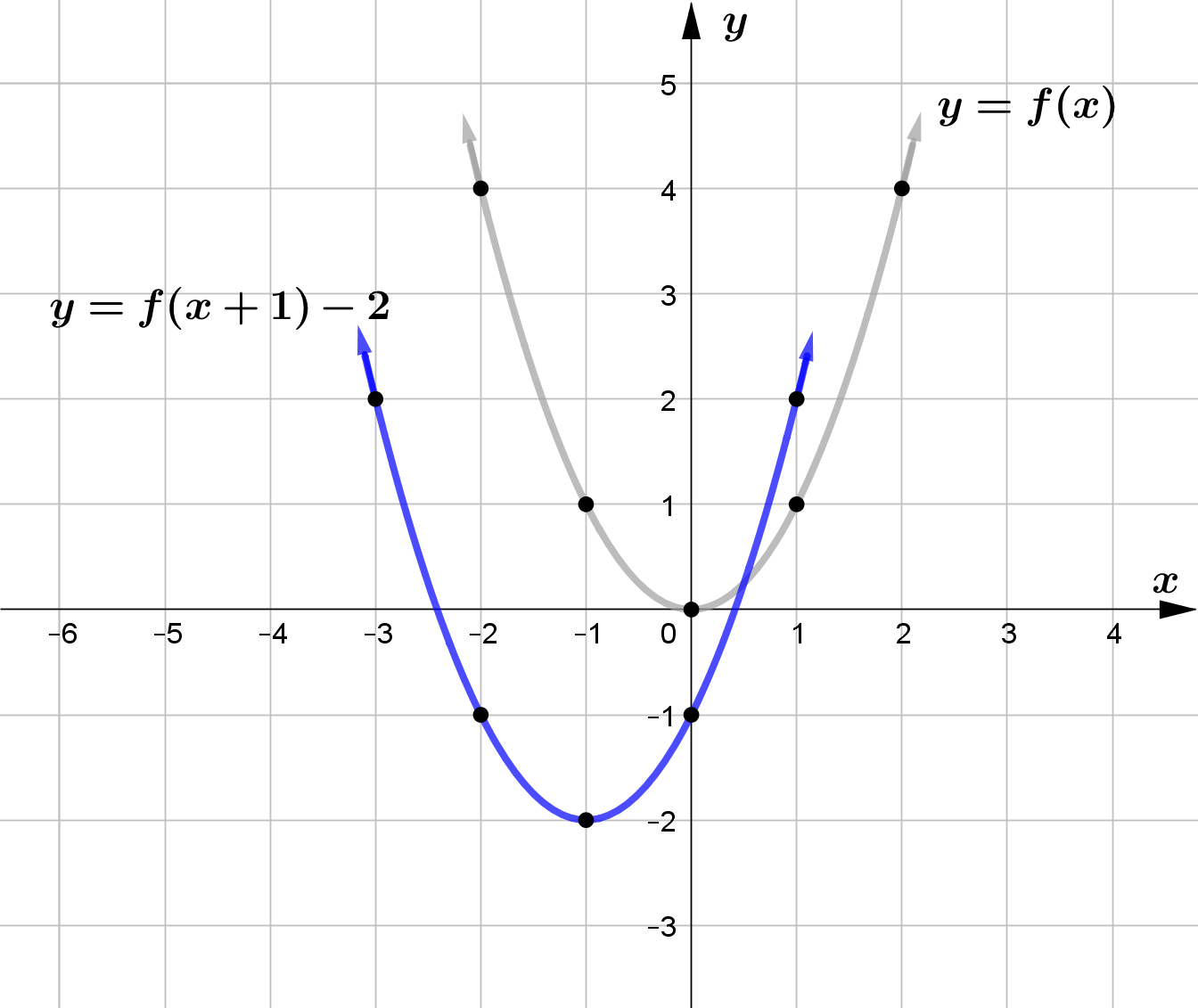
| (e) | $G(x)=f(x+1)−2$ |
| The grph of $y=G(x)$ can be obtained by shifting the grph of $y=f(x)$ 1 unit left followed by 2 units down. |
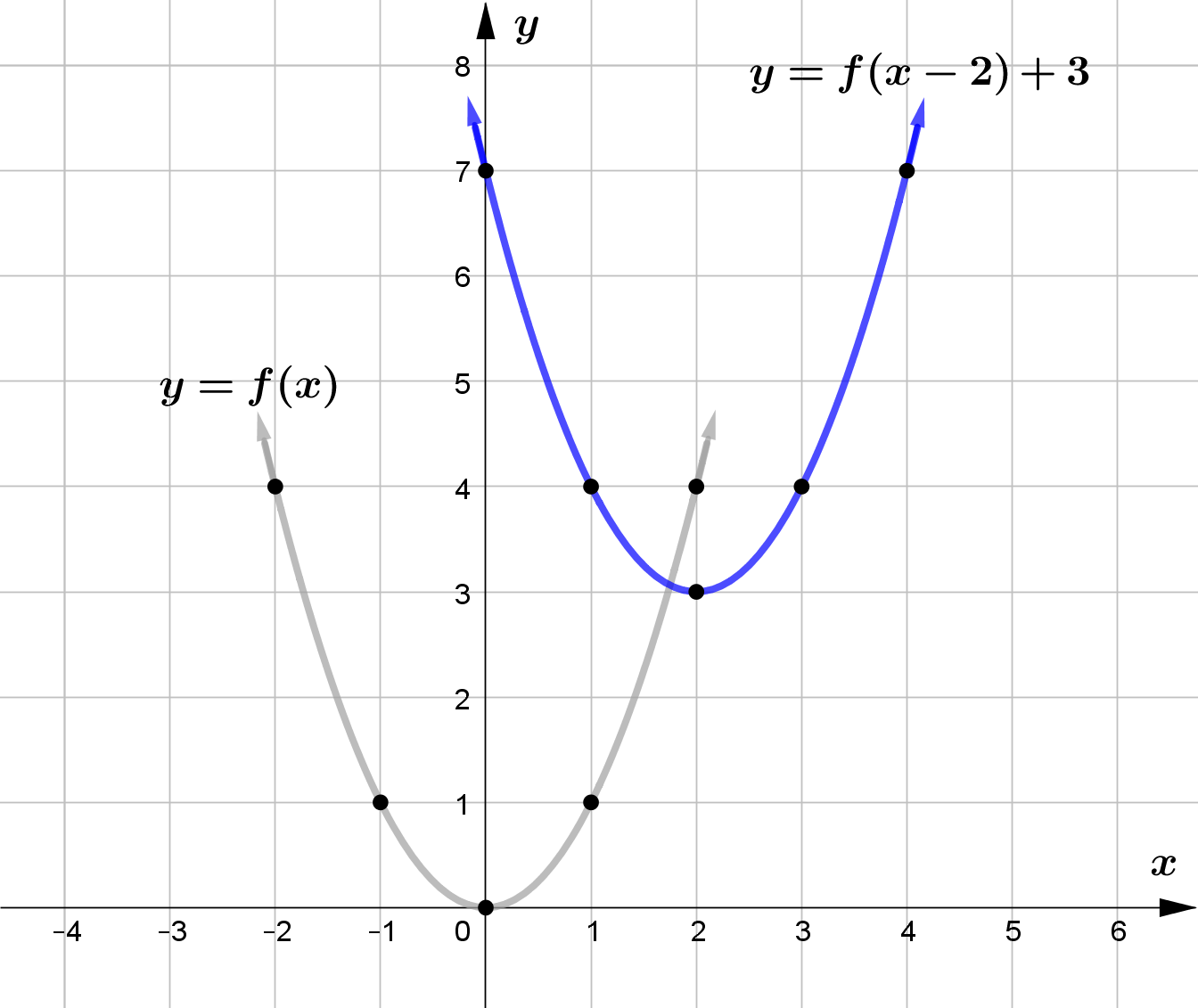
| (f) | $H(x)=f(x−2)+3$ |
| The grph of $y=H(x)$ can be obtained by shifting the grph of $y=f(x)$ 2 units right followed by 3 units up. |
Question (3)
Draw the graph $f(x) = |x|$. Hence using the transformations of $f(x)$, draw the graph of the following functions.
$\begin{array}{lll}
\text{(a) } g(x) = f(x) + 1 \\\\
\text{(b) } h(x) = f(x) - 3 \\\\
\text{(c) } p(x) = f(x-1) \\\\
\text{(d) } F(x) = f(x+3) \\\\
\text{(e) } G(x) = f(x+1) - 2 \\\\
\text{(f ) } H(x) = f(x-2) + 3
\end{array}$
SOLUTION
To sketch the graph of $y=x^2$, we should find some sample points on the graph.
$\begin{array}{|c|c|c|c|c|c|c|c|c|c|c|}
\hline x & -2 & -1 & 0 & 1 & 2\\
\hline y & 2 & 1 & 0 & 1 & 2\\
\hline
\end{array}$

| (a) | $g(x)=f(x)+1$ |
| The grph of $y=g(x)$ can be obtained by shifting the grph of $y=f(x)$ 1 unit up. |
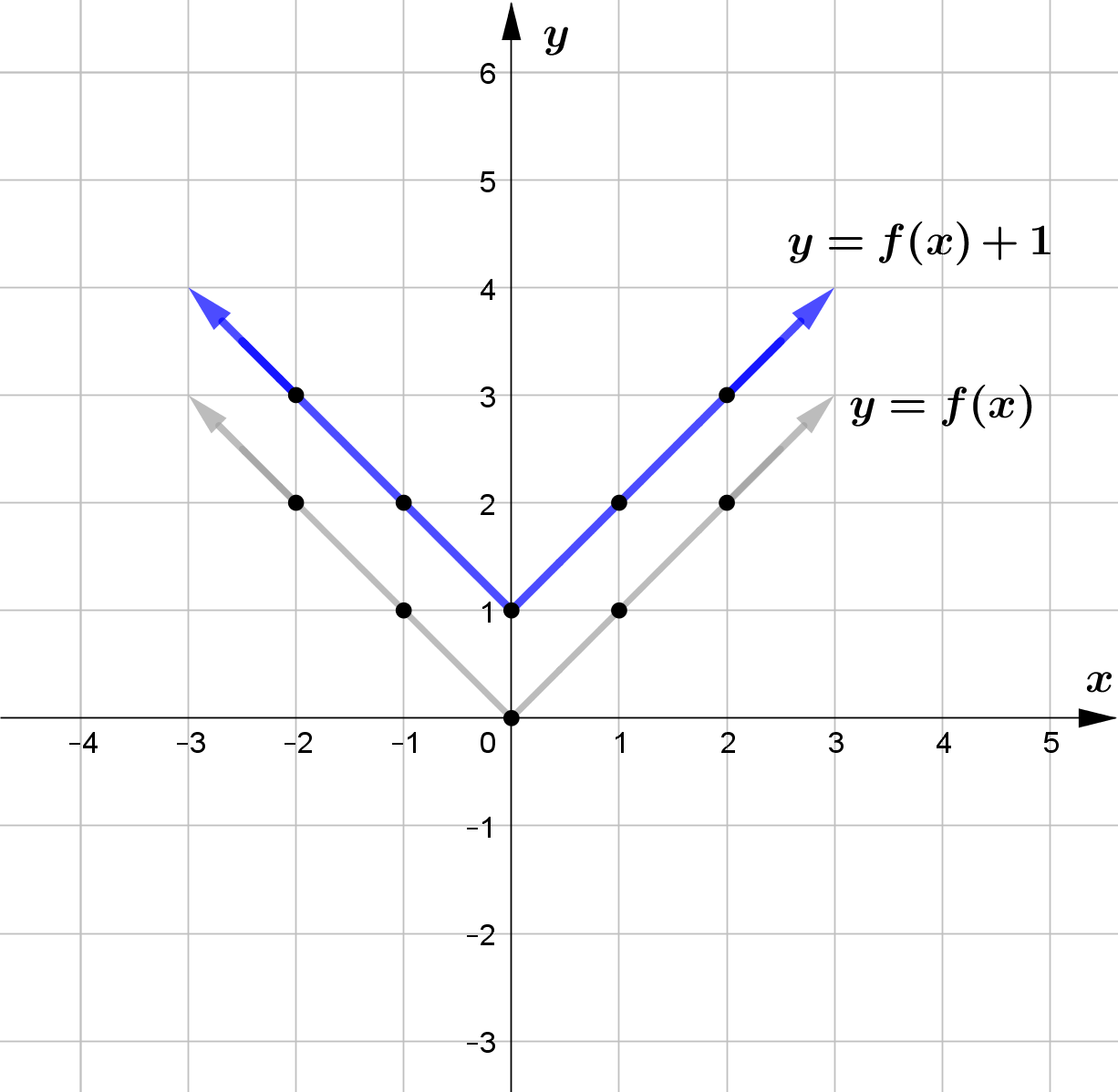
| (b) | $h(x)=f(x)−3$ |
| The grph of $y=h(x)$ can be obtained by shifting the grph of $y=f(x)$ 3 units down. |
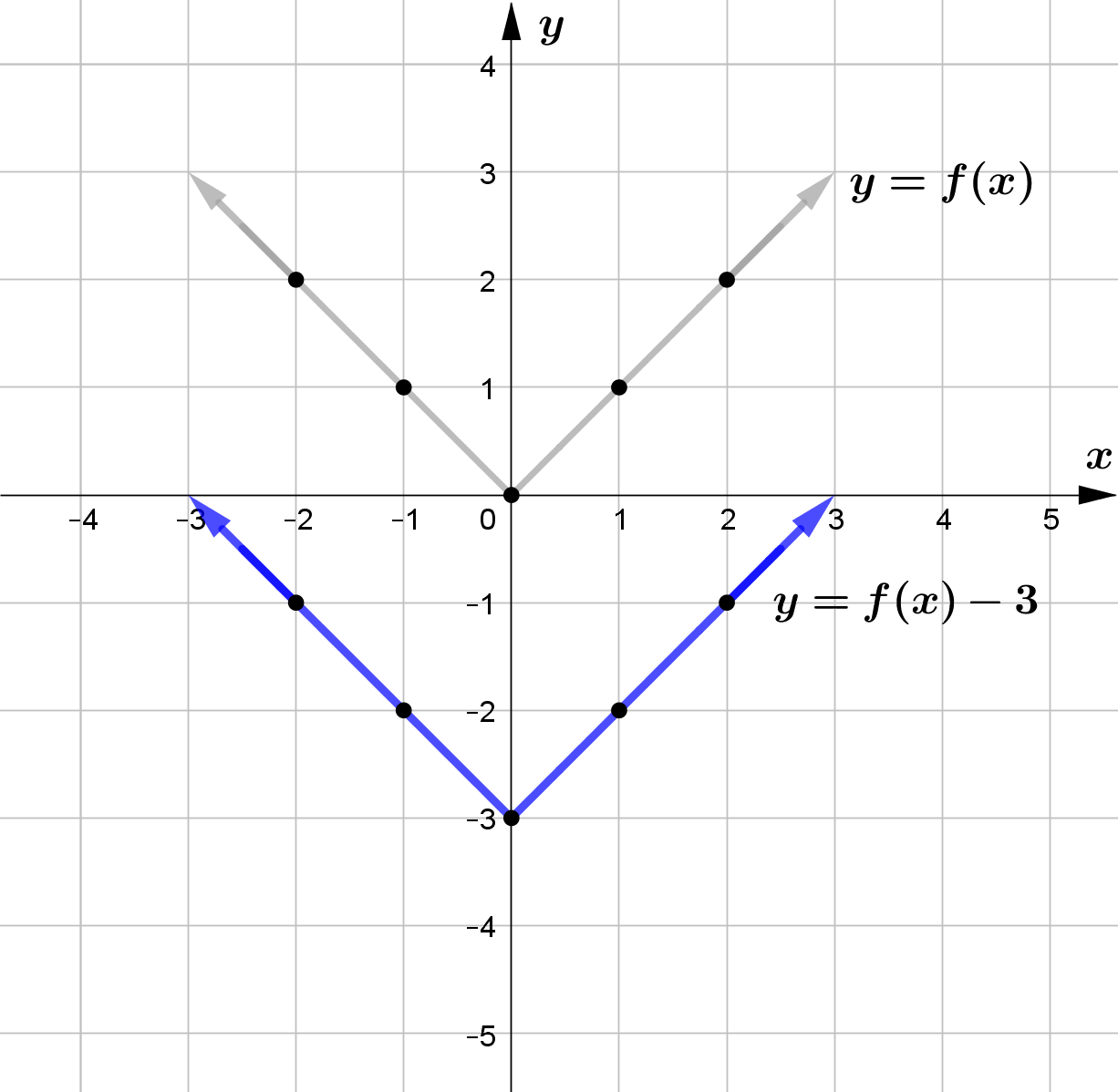
| (c) | $p(x)=f(x−1)$ |
| The grph of $y=p(x)$ can be obtained by shifting the grph of $y=f(x)$ 1 unit right. |
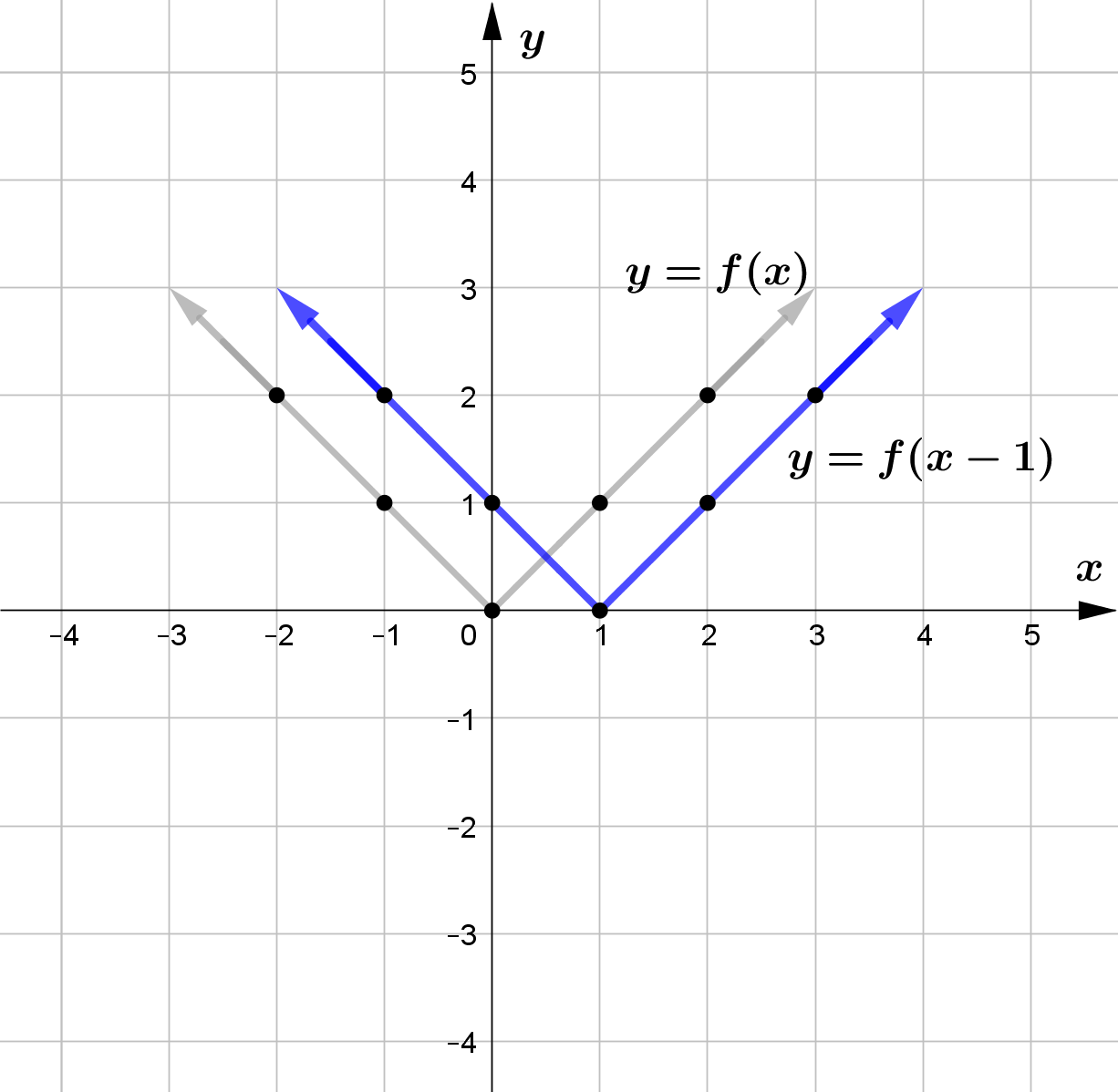
| (d) | $F(x)=f(x+3)$ |
| The grph of $y=F(x)$ can be obtained by shifting the grph of $y=f(x)$ 3 units left. |

| (e) | $G(x)=f(x+1)−2$ |
| The grph of $y=G(x)$ can be obtained by shifting the grph of $y=f(x)$ 1 unit left followed by 2 units down. |

| (f) | $H(x)=f(x−2)+3$ |
| The grph of $y=H(x)$ can be obtained by shifting the grph of $y=f(x)$ 2 units right followed by 3 units up. |
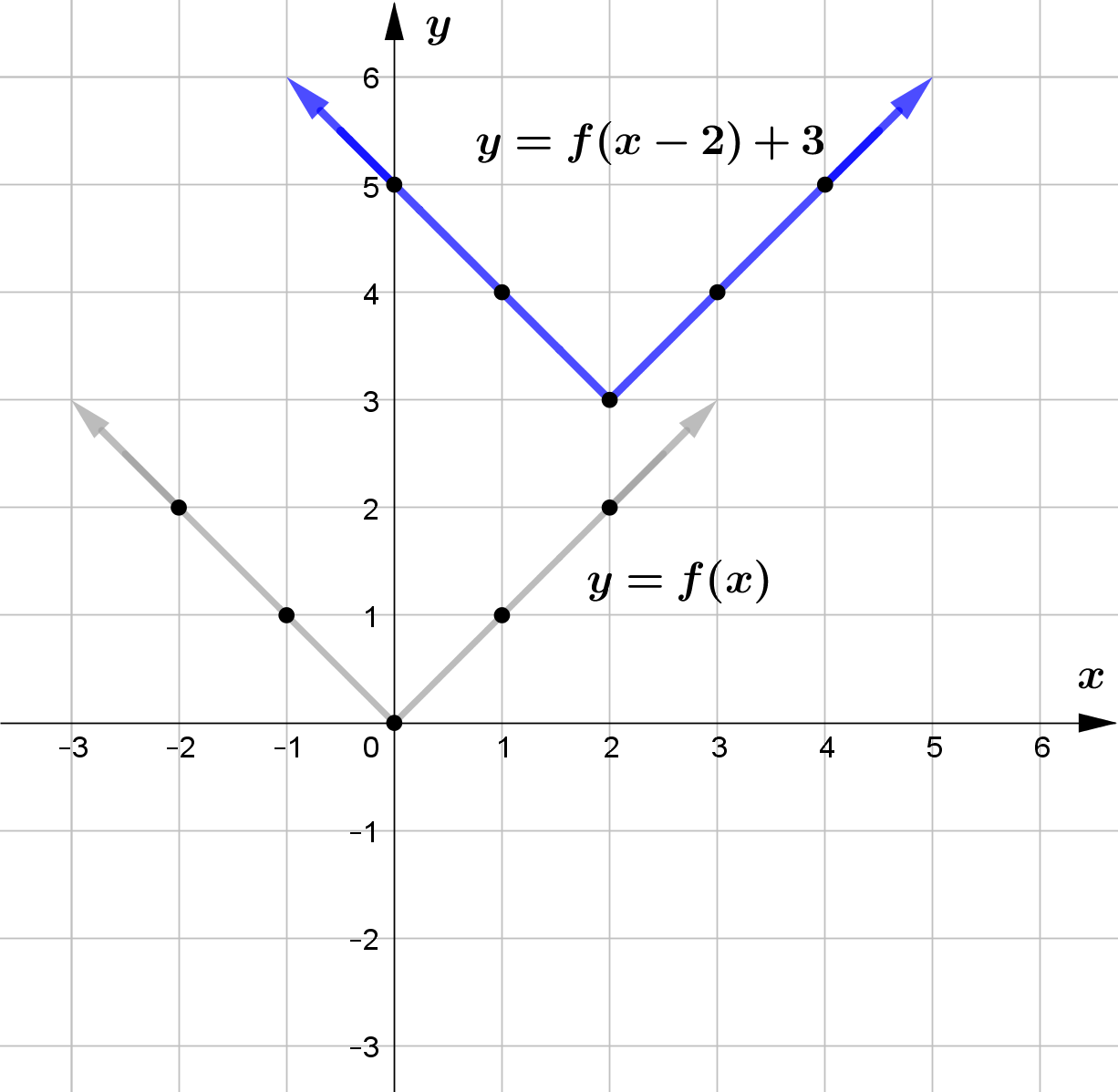
Question (4)
Draw the graph $f(x) = \sqrt{x}$. Hence using the transformations of $f(x)$, draw the graph of the following functions.
$\begin{array}{lll}
\text{(a) } g(x) = f(x) + 1 \\\\
\text{(b) } h(x) = f(x) - 3 \\\\
\text{(c) } p(x) = f(x-1) \\\\
\text{(d) } F(x) = f(x+3) \\\\
\text{(e) } G(x) = f(x+1) - 2 \\\\
\text{(f ) } H(x) = f(x-2) + 3
\end{array}$
To sketch the graph of $y=x^2$, we should find some sample points on the graph.
$\begin{array}{|c|c|c|c|}
\hline x & 0 & 1 & 4 \\
\hline y & 0 & 1 & 2 \\
\hline
\end{array}$
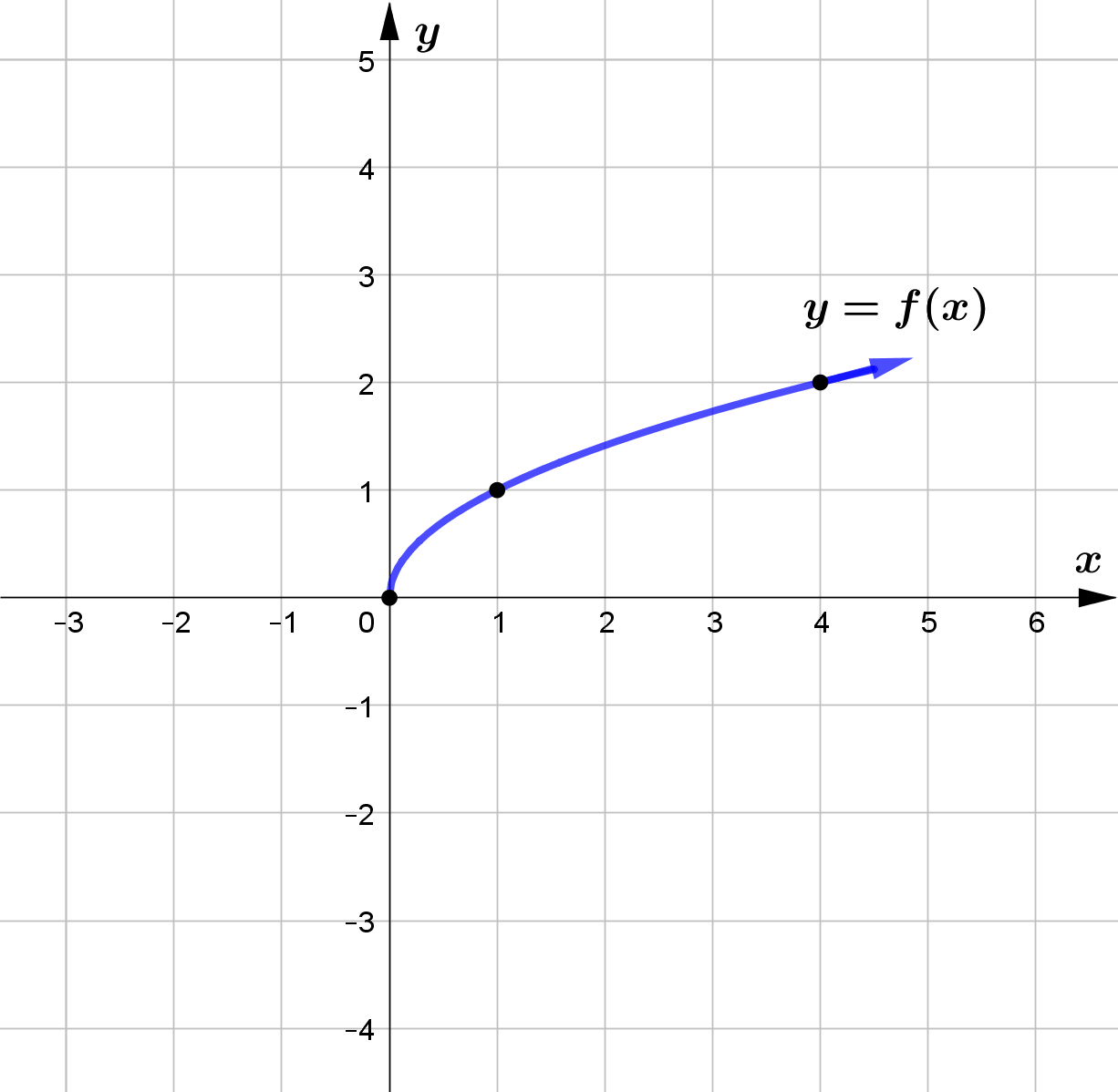
| (a) | $g(x)=f(x)+1$ |
| The grph of $y=g(x)$ can be obtained by shifting the grph of $y=f(x)$ 1 unit up. |
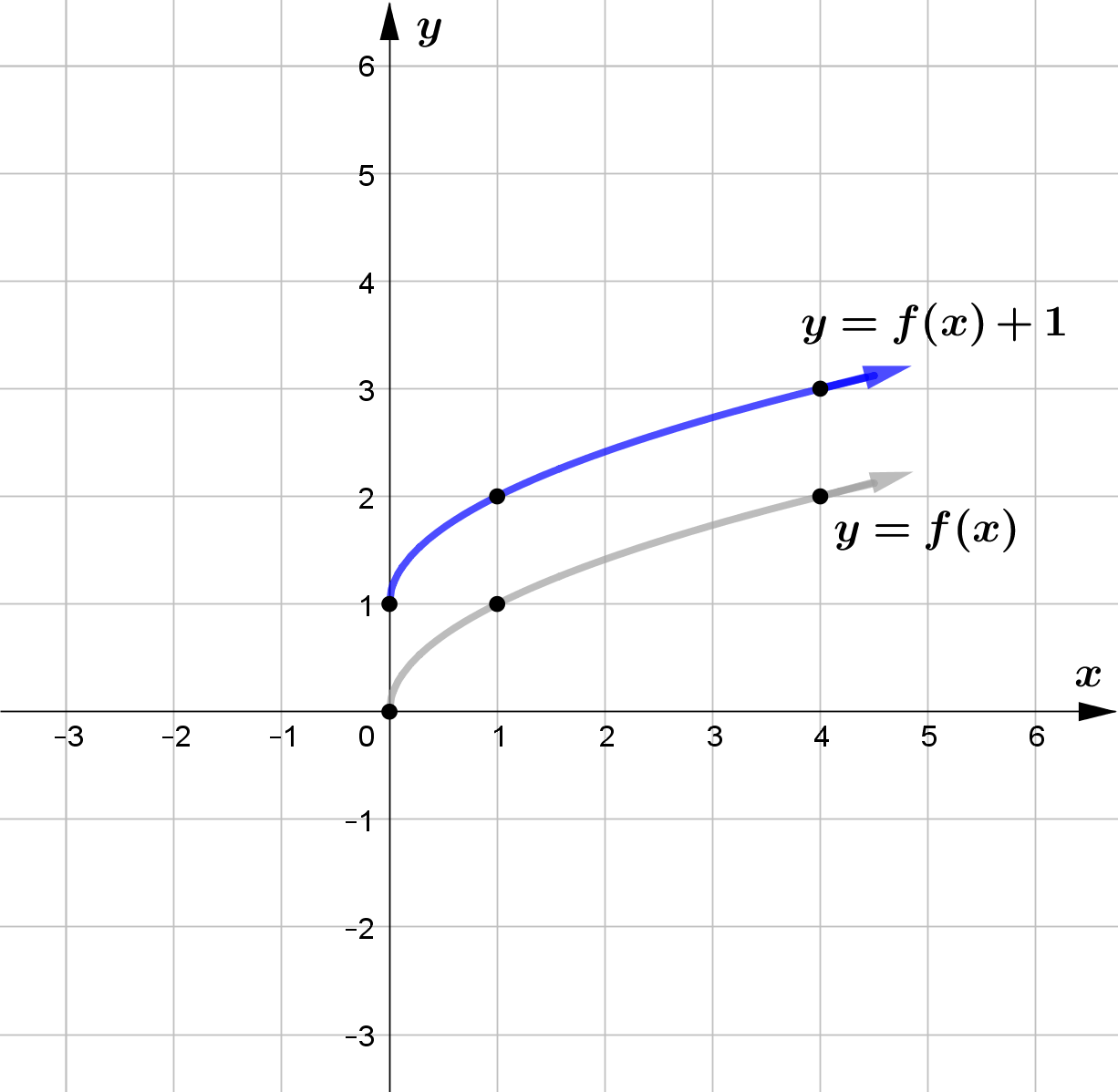
| (b) | $h(x)=f(x)−3$ |
| The grph of $y=h(x)$ can be obtained by shifting the grph of $y=f(x)$ 3 units down. |

| (c) | $p(x)=f(x−1)$ |
| The grph of $y=p(x)$ can be obtained by shifting the grph of $y=f(x)$ 1 unit right. |
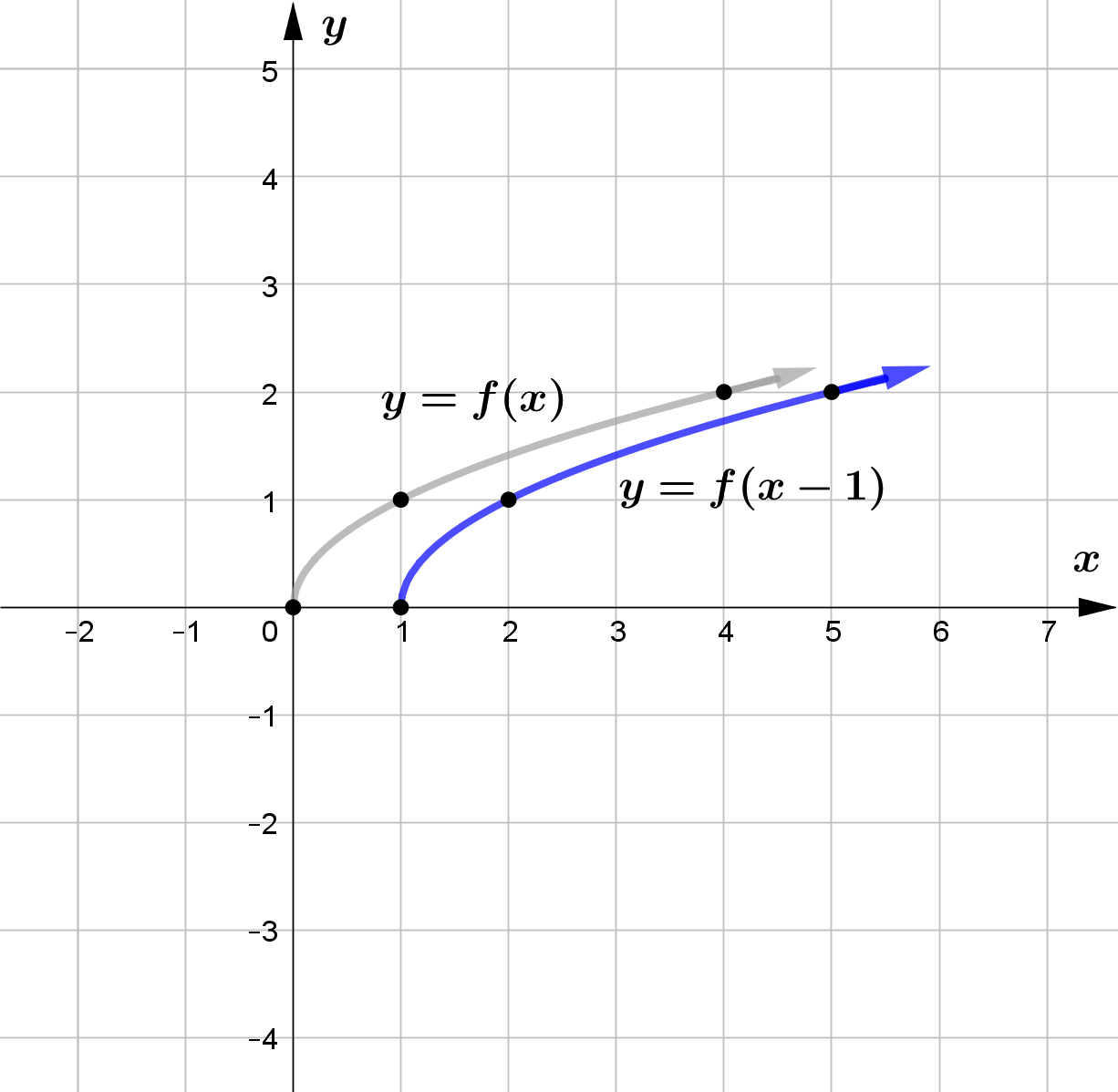
| (d) | $F(x)=f(x+3)$ |
| The grph of $y=F(x)$ can be obtained by shifting the grph of $y=f(x)$ 3 units left. |
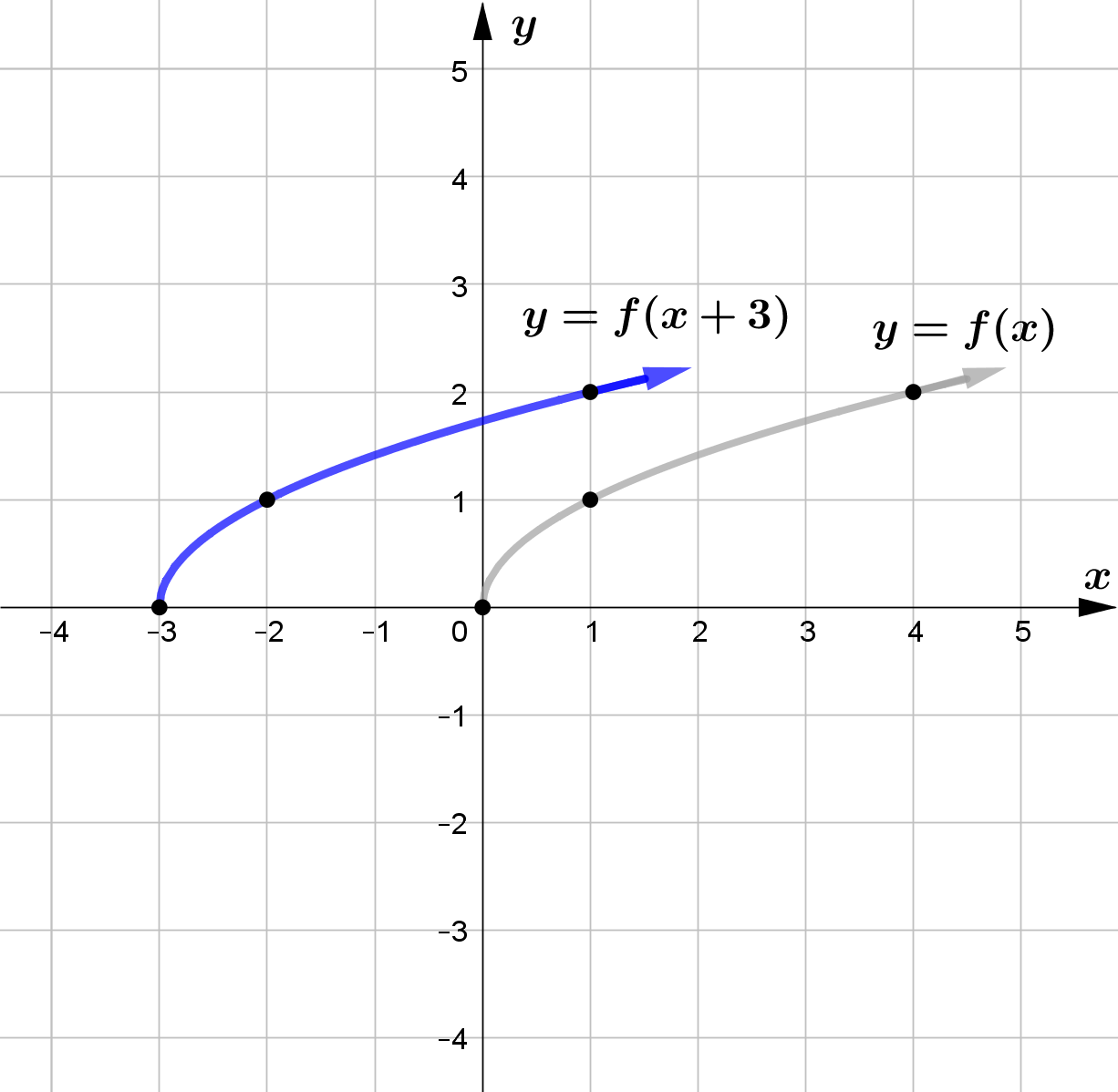
| (e) | $G(x)=f(x+1)−2$ |
| The grph of $y=G(x)$ can be obtained by shifting the grph of $y=f(x)$ 1 unit left followed by 2 units down. |
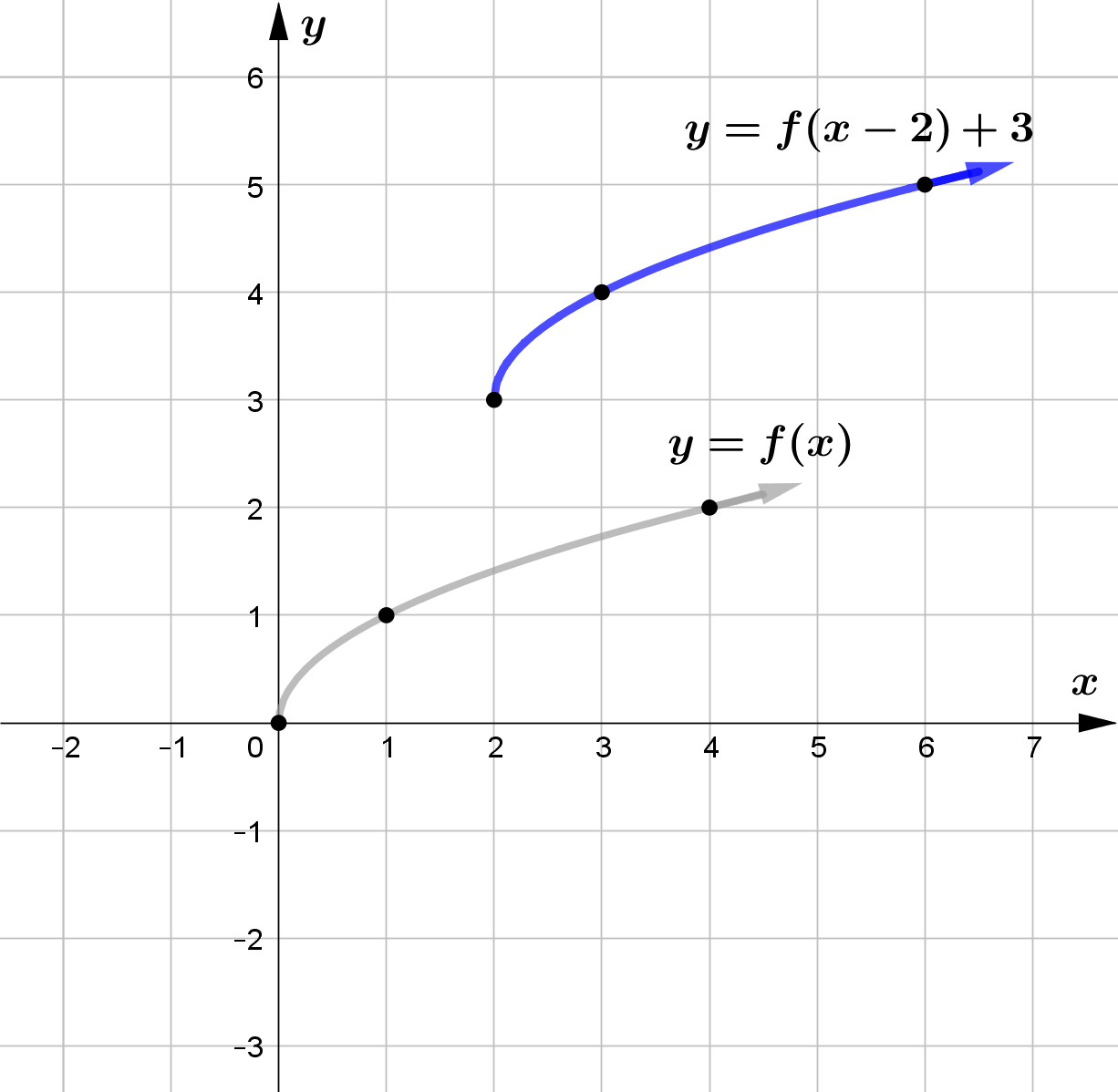
| (f) | $H(x)=f(x−2)+3$ |
| The grph of $y=H(x)$ can be obtained by shifting the grph of $y=f(x)$ 2 units right followed by 3 units up. |
Question (5)
If the graph of the quadratic function $f(x)$ has the vertex at $(-1,3)$, state the vertex after the given translations:
$\begin{array}{lll}
\text{(a) } f(x-2)+2\\\\
\text{(b) } f(x)-5 \\\\
\text{(c) } f(x+1)-3 \\\\
\text{(d) } f(x-6) \\\\
\text{(e) } f(x+1) - 2\qquad\quad \\\\
\text{(f) } f(x+2) + 1
\end{array}$
$\begin{aligned}
\text{(a) } & f(x-2)+2 \\\\
& (-1, 3)\rightarrow (-1+2, 3+2)=(1, 5)\\\\
\text{(b) } & f(x)-5 \\\\
& (-1, 3)\rightarrow (-1, 3-5)=(-1, -2)\\\\
\text{(c) } & f(x+1)-3 \\\\
& (-1, 3)\rightarrow (-1-1, 3-3)=(-2, 0)\\\\
\text{(d) } & f(x-6) \\\\
& (-1, 3)\rightarrow (-1+6, 3)=(5, 3)\\\\
\text{(e) } & f(x+1)-2 \\\\
& (-1, 3)\rightarrow (-1-1, 3-2)=(-2, 1)\\\\
\text{(f) } & f(x+2)+1 \\\\
& (-1, 3)\rightarrow (-1-2, 3+1)=(-3, 4)
\end{aligned}$
Question (6)
If the points $A(2,-3)$ lies on the graph of $y=f(x)$. Use transformation to find the map point of $A$
on the graph $y= g(x)$ such that
(a) $g(x) = f(x-2) + 1$.
(b) $g(x) = f(x+1) - \frac{1}{2}$.
Let the mapped point of $A(2, -3)$ be $A'(a,b)$.
$\begin{aligned}
&\\
\text{(a) } & \text{ After translation by } f(x-2)+1 \\\\
& a=2+2 = 4, b=-3+1=2\\\\
& \text{ The mapped point is } A'(4,2)\\\\
\text{(b) } & \text{ After translation by } f(x+1) -\frac{1}{2} \\\\
& a=2-1 = 1, b=-3-\frac{1}{2}=- \frac{7}{2}\\\\
& \text{ The mapped point is } A'\left(1,-\frac{7}{2}\right)\\\\
\end{aligned}$
Question (7)
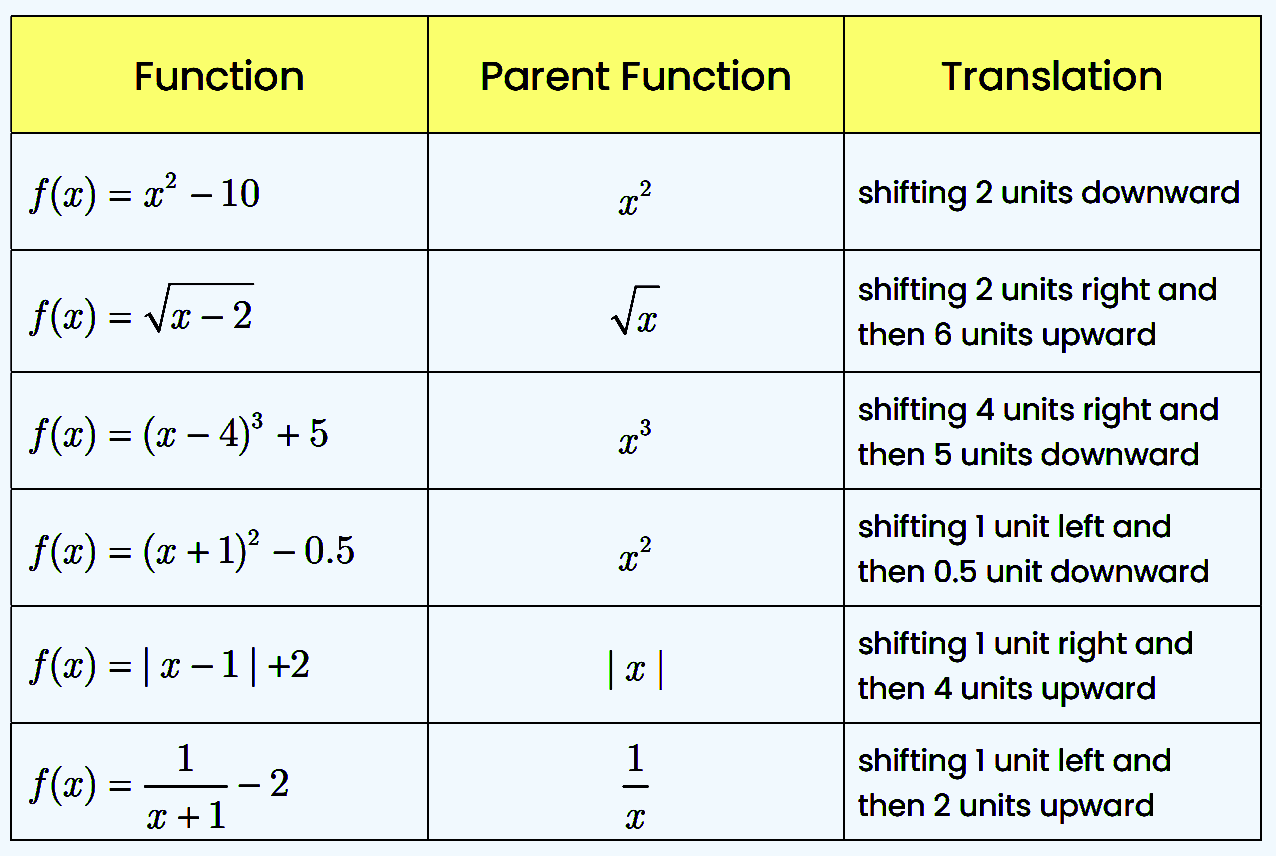
State the parent function and the translation that is occurring in each of the following functions.
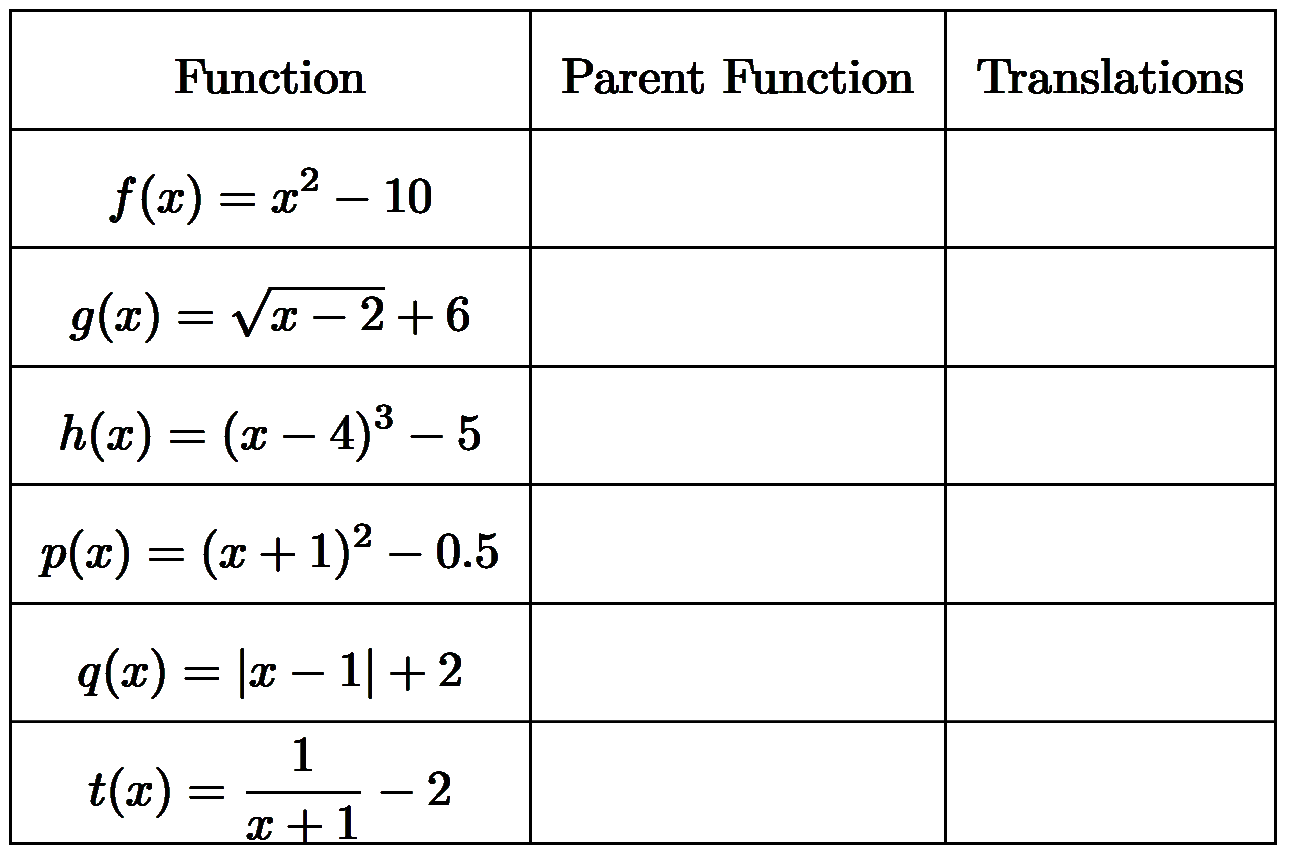
SOLUTION

Post a Comment for "Transformation of Functions: Translation"